To find the coefficient of x 7 y 2 in the expansion of (x 2y) 9 Formula Used A general term, T r1 of binomial expansion (x y) n is given by, Now, finding the general term of the expression, (x 2y) 9, we get The value of r for which coefficient of x 7 y 2 is defined R = 2 About this tutor › About this tutor › (xy)3expanded has 4 terms, 1 more than the exponent, x3x2y xy2and y3 x is decreasing from 3 to 0 from left to right, as y increases from 0 to 3 Any number or variable to the 0 power is 1 Then you need the coefficients for each of the 4 termsFind the number of terms in the expansion of x y 3 x y 3 2 x y 3 x y 3 2 = x 2 y 2 3 2 = x 2 y 2 6No of terms in the expansion = n

How Can We Expand X Y 1 2 Youtube
(x+y-1)^3 expansion
(x+y-1)^3 expansion- Find the coefficient of x^5y^7 in the expansion of (5x2y)^12 12 (x)^5 (2y)^7 3 My answer which i got wrong is 2x^5(128y^7)= x^5y^7 Physics A steel wire, 150 m long at 10°C, has a coefficient of linear expansion of 11 ´ 106/C° GiveMartin Berz , in Advances in Imaging and Electron Physics, 1999 541 Differentiating ODE Solvers Intuitively, the most direct method for obtaining Taylor expansions for the flow of an ODE is to recognize that a numerical ODE solver describes a functional dependency between initial conditions and final conditions Thus, by replacing all arithmetic operations in it by the corresponding ones




Section 9 Binomial Expansion Questions About Homework Submit Homework Recall The Exercises We Did Last Class 04 01 19 Math 106 Section 9 1 Slideshow And Powerpoint Viewer What Is Binomi
Show, by left side, that $$\frac{x^3y^3}{xy} = x^2xyy^2,$$ or $$\frac{x^3y^3}{x^2xyy^2} = xy$$ You may read about "Long Division of Polynomials" See also LINK for knowing the processHere is a music track from the exclusive FZero X EXpansion Kit This time, it is a new track called "Rollercoaster", which is found in "Sand Ocean 3" and "The formula is (xy)³=x³y³3xy(xy) Proof for this formula step by step =(xy)³ =(xy)(xy)(xy) ={(xy)(xy)}(xy) =(x²xyxyy²)(xy) =(xy)(x²y²2xy
In the denominator for each term in the infinite sum HistoryExpand (x y z)^10 Extended Keyboard; Expand (1/xy/3)^3 solve it fastly density1 density1 Math Secondary School answered Expand (1/xy/3)^3 solve
The base is X;The exponent is two;In elementary algebra, the binomial theorem describes the algebraic expansion of powers of a binomial According to the theorem, it is possible to expand the polynomial n into a sum involving terms of the form axbyc, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positive integer depending on n and b For example, 4 = x 4 4 x 3 y 6 x 2 y 2 4 x y 3 y 4 {\displaystyle ^{4}=x^{4}4x^{3}y6x^{2}y
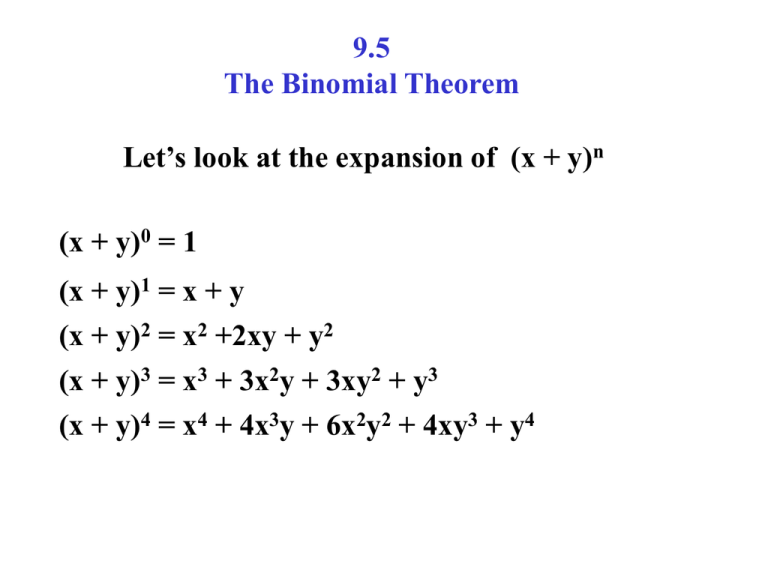



9 5 The Binomial Theorem



Expansion And Factorisation Bingo Card
Expand (x1)^3 Expand Calculator Symbolab This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more Accept Solutions Graphing Practice Geometry betaIn the expression, if we replace y with (− y), we will get the identity x 3 − y 3 Now, let's further verify this numerically with an example To verify, let's take the values for x and y and put in the LHS and RHS of the identitySolution Expanding the binomial using the binomial theorem formula, we have Therefore, the coefficient for x4y3 x 4 y 3 is −25 − 25




What Is The Formula Of Math A B 3 Math Quora




16 Pokemon Xy Generations Expansion Set Caterpie 3 5e9 Ebay
Answer to How to expand x^3y^3? Example 7 Find the coefficient of x6y3 in the expansion of (x 2y)9 We know that General term of expansion (a b)n is Tr1 = nCr an–r br For (x 2y)9, Putting n = 9 , a = x , b = 2y Tr 1 = 9Cr (x)9 – r (2y)r = 9Cr (x)9 – r (y)r (2)r We need to find coefficient of x6 y3 Comparing yr = y3 r = 3 Putting r = 3 in (1) T31 = 9C3 x91 2 1 for n = 2 the x^2 term is the rightmost one here so we'll get 1 times the first term to the 0 power times the second term squared or 1*1^0* (x/5)^2 = x^2/25 so not here 1 3 3 1 for n = 3 Squared term is second from the right, so we get 3*1^1* (x/5)^2 = 3x^2/25 so not here 1




Learn Algebraic Identity Of X Y And X Y In 3 Minutes




Don T Understand Why This Binomial Expansion Is Not Valid For X 1 Mathematics Stack Exchange
We know that (xy) 3 can be written as (xy)(xy)(xy) We know that (xy)(xy) can be multiplied and written as x 2xy yx y 2 (xy) = x 22xy y 2 (xy) = x 32x 2 y xy 2yx 2 2xy 2y 3 = x 33x 2 y 3xy 2y 3 Answer (xy) 3 =x 33x 2 y 3xy 2y 3Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, scienceX^3 y^3 z^3 3x^2y 3xy^2 3x^2z 3z^2x 3y^2z 3z^2y 6xyz Lennox Obuong Algebra Student Email obuong3@aolcom




Learn Algebraic Identity Of X Y And X Y In 3 Minutes
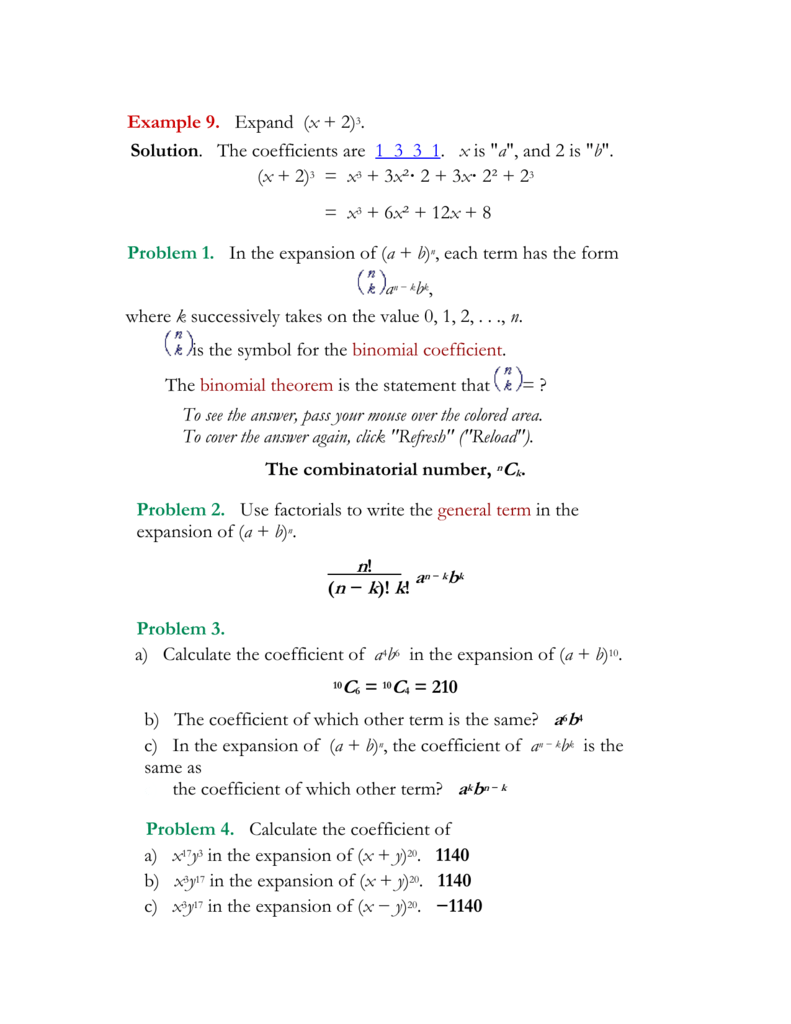



Alg2 March28 The Answers
Binomial Expansions Binomial Expansions Notice that (x y) 0 = 1 (x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 3 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4 Notice that the powers are descending in x and ascending in yAlthough FOILing is one way to solve these problems, there is a much easier wayMassimiliano The rule is \displaystyle{\left({a}{b}\right)}^{{3}}={a}^{{3}}{3}{a}^{{2}}{b}{3}{a}{b}^{{2}}{b}^{{3}} So \displaystyle{\left({x}{2}{y}\right)}^{{3}}={x}^{{3}}{3}{\left({x}\right)}^{{2}}{\left({2}{y}\right)}{3}{x}{\left({2}{y}\right)}^{{2}}{\left({2}{y}\right)}^{{3}}=TAYLOR SERIES Expand x^2y3y2 in the powers of x1 and y2 up to 3 degree terms Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try




16 Pokemon Xy Steam Siege Expansion Set Base 3 Hoppip
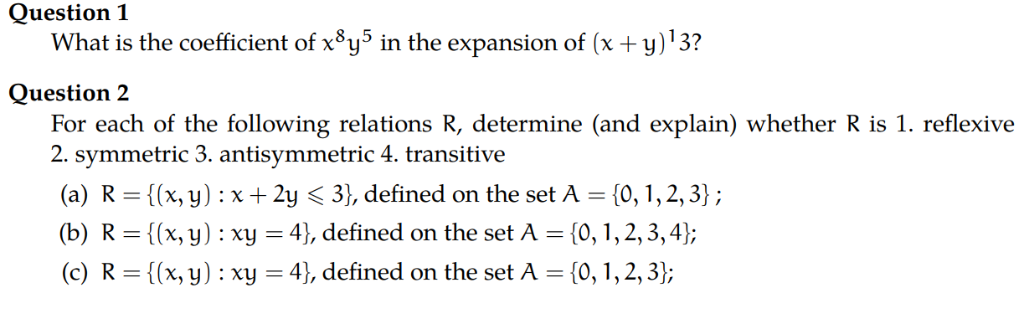



Question 1 What Is The Coefficient Of X8y5 In The Chegg Com



No comments:
Post a Comment